Preparation Manual
Section 5: Sample Constructed-Response Question Early Childhood: PK–3 (292)
General Directions
This question requires you to demonstrate your knowledge of the subject area by providing an in-depth written response. Read the question carefully before you begin to write your response to ensure that you address all components. Think about how you will organize what you plan to write.
The final version of your response should conform to the conventions of standard English. Your written response should be your original work, written in your own words, and not copied or paraphrased from some other work. You may, however, use citations when appropriate.
Exhibits for the constructed-response question will be presented in a tabbed format on the computer-administered test. You will have the ability to move between exhibits by clicking on the tab labels at the top of the screen.
An on-screen answer box will be provided on the computer-administered test. The answer box includes a white response area for typing your response, as well as tools along the top of the box for editing your response. A word counter that counts the number of words entered for the response is also provided in the lower left corner of the box. Note that the size, shape, and placement of the answer box will depend on the content of the assignment.
Sample Assignment
Use the information in the exhibits to complete the assignment that follows.Using your knowledge of mathematics content and pedagogy, analyze the information provided and write a response of approximately 400 to 600 words in which you:
- describe one area of academic need that the student demonstrates related to the foundational mathematics skill or objective specified;
- identify and describe a developmentally appropriate, effective instructional strategy or intervention to address the student's identified need in the mathematics skill or objective;
- explain why this instructional strategy or intervention would be effective, using sound reasoning and knowledge of foundational mathematics skills;
- describe a developmentally appropriate method of informal assessment to effectively monitor the student's progress toward the identified skill or objective; and
- describe one cross-curricular activity that could be integrated into the curriculum to support learning or extension of the identified foundational mathematics skill or objective.
Lesson Description
In the middle of the school year, a third-grade class has developed an understanding of multiplication facts. The class is currently working on a lesson objective of applying multiplication knowledge to solve and explain the solution for one- and two-step word problems using numbers and pictures, in accordance with the following standards from the Texas Essential Knowledge and Skills (TEKS) for Grade 3 Mathematics.
|
§111.5 Mathematics Grade 3 number and operations. 4. The student applies mathematical process standards to develop and use strategies and methods for whole number computations in order to solve problems with efficiency and accuracy. The student is expected to: (K) solve one-step and two-step problems involving multiplication and division within 100 using strategies based on objects; pictorial models, including arrays, area models, and equal groups; properties of operations; or recall of facts. |
During the independent practice portion of the teacher's lesson, students solve differentiated word problems based on real-world scenarios, according to their level of understanding of multiplication facts. Students have been instructed to share how they solved the problems with a partner who is working on the same set of differentiated problems and draw a picture to represent their work. After 10 minutes, the teacher notices that one student, Benjamin, has finished and is waiting for his partner to finish.
Name: Benjamin Score: 100% |
||
| 5 times 5 equals 25 | 1 times 2 equals 2 | 9 times 6 equals 54 |
| 6 times 7 equals 42 | 3 times 3 equals 9 | 4 times 8 equals 32 |
| 7 times 6 equals 42 | 8 times 0 equals 0 | 3 times 3 equals 9 |
| 4 times 1 equals 4 | 7 times 7 equals 49 | 5 times 6 equals 30 |
| 0 times 9 equals 0 | 6 times 3 equals 18 | 2 times 2 equals 4 |
| 9 times 9 equals 81 | 5 times 1 equals 5 | 7 times 3 equals 21 |
| 8 times 3 equals 24 | 4 times 7 equals 28 | 6 times 6 equals 36 |
| 2 times 4 equals 8 | 5 times 9 equals 45 | 8 times 6 equals 48 |
| 7 times 8 equals 56 | 0 times 1 equals 0 | 7 times 6 equals 42 |
| 3 times 9 equals 27 | 8 times 4 equals 32 | 2 times 7 equals 14 |
|
1. Tashina is setting up rows of chairs for a school concert. She makes 8 rows, and puts 5 chairs in each row. How many chairs does she set up? Benjamin has written a row of 8 Xs, and under that a row of 5 Xs. Benjamin has then written, 8 plus 5 equals 13 and under that 13 chairs. |
|
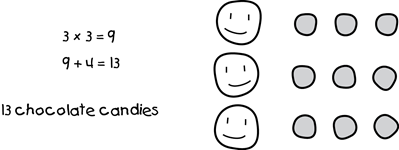
2. Naomi has a bag of cookies she wants to share with 3 friends. She wants each friend to get 3 cookies. Each cookie has 4 chocolate candies on top. How many chocolate candies are there in all?
|
|
3. Rosie has 8 boxes with 7 crayons in each box. Ethan has 7 boxes with 8 crayons in each. Who has more crayons, Rosie or Ethan? Why? Rosie has more crayons because she has more boxes. |
Excerpt of Conversation
Teacher: Now you won't have to wait so long to talk about how you found your answers. Can you tell me how you came up with your equation for the first problem?
Benjamin: (points to the first problem) In the problem I saw that there was an 8 in the first row, and 5 in the second row, so I added 8 and 5, which is 13. That must mean Tashina has 13 chairs.
Teacher: And for the second problem?
Benjamin: Well, here I saw that Naomi has cookies to give to 3 friends, and each friend gets 3 cookies. This sounds like equal sharing, so I multiplied 3 times 3 to get 9. Then, she sees that there are 4 chocolate candies on top of each cookie. 9 + 4 is 13. So, there are 13 chocolate candies.
Teacher: You said sharing cookies with friends sounds like equal sharing. Can you explain what you mean by that?
Benjamin: This sounds like when we were practicing multiplication by adding over and over, so it made me think I should multiply.
Teacher: That's a good connection. What happened when you went to solve the last problem?
Benjamin: Oh, I saw right away that Rosie has more boxes of crayons than Ethan does, so she has more crayons!
Sample Responses and Rationales
Score Point 4
Benjamin demonstrates a lack of foundational skills for applying multiplication concepts in word problems as specified in the objective and the TEKS (Exhibit 4). He has demonstrated mastery of automaticity in multiplication facts, as shown in the "Mathematics Fact Timed Test" (Exhibit 5), where he scored 100%. However, Benjamin was unable to give evidence of his understanding of multiplication concepts when solving one- and two-step word problems in the Independent Practice Activity (Exhibit 6). In the first problem, Benjamin created an addition equation rather than a multiplication equation. He did not identify the mathematics vocabulary necessary to solve the word problem. Therefore, he struggled with creating an array to fully illustrate how to solve the problem. In the second problem, he created an array, but again used an addition equation to illustrate one of the steps rather than multiplication, which resulted in an incorrect answer. In the third problem, Benjamin did not create a picture, array, or an equation. He relied on an incorrect interpretation of vocabulary and solved the problem incorrectly.
A developmentally appropriate instructional strategy the teacher could implement would be a "Think Aloud," verbalizing thoughts while teaching a logical process, using the mnemonic device "CUBES." The teacher would read the problem with Benjamin, circle the numbers, underline the question, box the key words, evaluate and draw, then solve and check (circle, underline, box, evaluate, solve). In the first problem, while thinking aloud, they would circle 8 and 5 and underline the question. They would box "chairs in each row". Next, the teacher would demonstrate using manipulatives to create an array, which is a concrete representation of the multiplication problem. They would create 8 rows of 5 using manipulatives and a drawing. Lastly, they would determine the correct operation to solve the problem. They could discuss that addition could be used, but it would need to be a multi-step problem. They could also discuss how multiplication is a way to do addition more quickly. They would then write the equation to solve the problem. The teacher would model additional word problems, guiding Benjamin in the steps. Finally, Benjamin will solve problems on his own.
This strategy is effective because direct, explicit instruction in the use of step-by-step problem-solving with manipulatives, pictures, and writing the equation is a progressive process, moving from the concrete to the abstract. The teacher is modeling the process which will provide Benjamin the opportunity to understand the steps involved in solving the problem successfully. Practicing solving word problems using this strategy consistently, Benjamin will begin to see similarities in other problems. This practice illustrates the Gradual Release of Responsibility model (I do, we do, you do).
The teacher can monitor Benjamin's progress with a checklist and anecdotal notes based on regular observation of Benjamin's work. The checklist should include specific steps for Benjamin to follow to solve word problems using CUBES. The teacher's observations of and discussions with Benjamin about his problem-solving approach will allow the teacher to verify mastery and make necessary adjustments to instruction.
To support and extend learning, the teacher could ask Benjamin to write his own mathematics story problems. The teacher could provide a template with characters, conflict, and resolution. This activity could strengthen Benjamin's problem-solving skills by giving him an opportunity to apply mathematics vocabulary and apply his knowledge of multiplication concepts through the story elements of a mathematics word problem. He could share the story with peers and ask them to solve the problem. He can verbalize how to use the steps to solve the equation; thus, providing more reinforcement. This extension lesson integrates math vocabulary, reading, writing, and speaking.
Rationale for the Score of 4
This response reflects a thorough understanding of the relevant content knowledge and skills. The response fully addresses all parts of the assignment and demonstrates an accurate, highly effective application of the relevant content knowledge and skills. The response provides strong, relevant evidence; specific examples; and well-reasoned explanations.
Completion: Notice that each of the five tasks presented in the assignment are answered completely, and in the order presented in the prompt. The response fully described and explained Benjamin's academic need citing relevant evidence from the exhibits provided. The candidate fully described a developmentally appropriate instructional strategy that effectively targets Benjamin's needs using professional knowledge and in a sequential, logical order. The response then offers a sound rationale of why the strategy will be effective, which reflects appropriate pedagogical knowledge of teaching mathematics to early childhood students. An informal assessment to effectively monitor Benjamin's progress toward the skill is thoroughly described. The candidate described a cross-curricular activity that could be integrated into the curriculum as an extension.
Application of Knowledge: As you read the response, note the accurate, current application of professional knowledge about teaching young children mathematics. The candidate interpreted the data provided accurately to identify Benjamin's need, used that information to design an appropriate strategy to address his needs, explained why the strategy would be effective using professional knowledge pedagogy, and continued the skills into another lesson via cross-curricular integration. The teacher is explicitly modeling each step through the "Think Aloud." Manipulatives, pictorial representation, and sequential learning are developmentally appropriate and relevant to addressing Benjamin's academic need of understanding and applying multiplication concepts to solve word problems. Checklists combined with anecdotal notes are effective progress monitoring tools. The extension strategy incorporates other curricular areas.
Support: The response supports assertions with specific, relevant evidence from the exhibits provided. The strategy, rationale, progress monitoring, and extension activity have specific examples. The response cites evidence from the exhibits provided. The strategy is clearly presented with specific supporting details for each step. The rationale for the strategy's effectiveness reflects sound reasoning. The extension lesson has specific examples.
Score Point 2
One area of academic need that the third grade student, who is working on his math skills, has is how to solve multiplication word problems. On the practice activity, Benjamin didn't answer any of the questions correctly. He substituted addition for multiplication and couldn't explain to his teacher how he solved his problems. He drew some pictures and one array but still was not able to correctly solve the problems. When the teacher was talking with him, it seemed like Benjamin knew how to multiply, but he also seems confused on the difference in multiplying, dividing, adding, and subtracting.
The teacher should instruct Benjamin in drawing pictorial arrays to represent the information he needs to solve each word problem. Benjamin drew eight X's to represent eight rows, but he did not draw five chairs in each row. If the teacher provided manipulatives or some other kind of real world tool, Benjamin would be better able to draw pictorials. She could have even used her classroom to help him understand. How many rows of desks? How many desks in each row? Then Benjamin would have a real world connection.
Exit tickets are a very effective way to formatively assess students' knowledge. The teacher can create exit tickets with word problems and then Benjamin would complete one ticket each day. He could have a chart with each day and then each time he was correct, he could put a sticker or check mark. At the end of the week, the teacher could have an incentive to reward him for getting at least 3 correct. By offering an incentive like station time, Benjamin will definitely increase his effort and ultimately his success. Since the problem he is working on is about cookies, the teacher could use toy cookies or pictures of real cookies to make it more of a real world math problem.
The teacher can work with other teachers on her team to create cross curricular activities. This could be in science, art, language arts and physical education. The PE teacher could have the students make life size arrays, in art they could create pictures of arrays with paints, and so forth. There are so many ways to make real world connections for our students. Creating real world activities to help Benjamin understand the math problems will increase his success rate.
Rationale for the Score of 2
The "2" response reflects a limited understanding of the relevant content knowledge and skills. The response partially addresses some of the parts of the assignment and demonstrates a limited application of the relevant content knowledge and skills. The response provides limited evidence and examples or explanations, when provided, are only partially appropriate.
Completion: Notice that the response does not fully respond to each portion of the assignment. The response describes an academic need, but unlike a score point "4" or "3" response, the evidence cited is presented in a less specific manner with partial evidence from the exhibits. The response provides an instructional strategy without the specifics of how to implement the strategy reflecting limited pedagogical knowledge of teaching mathematics to early childhood students. The response partially describes an informal assessment and a cross-curricular activity. This differs from the score point "4" or "3", which would have fully responded to each part of the assignment with more specificity and strong pedagogical knowledge.
Application of Knowledge: As you read the response, note the lack of accurate, current application of professional knowledge about teaching young children mathematics. The response demonstrates a partially accurate, limited application of the relevant content knowledge and skills. The response identifies a need related to multiplication, but the response does not explain why the strategy would be effective. This response begins with some information from the exhibits to determine an academic need. The academic need is partially identified. A strategy to address the need is provided, however, the response lacks a clear description of how the strategy would be implemented, the steps the teacher would take, and the order of how the instruction would be presented. It focuses on helping Benjamin make real-world connections, which is important, but does not address how the teacher will specifically instruct Benjamin to draw the arrays and then apply that knowledge to solve multiplication word problems. The informal assessment focuses on rewards and incentives for motivation and real-world connections and not on the more relevant, important information. The response demonstrates limited content knowledge regarding creating appropriate assessments to monitor student progress. This differs from the score point "4" or "3" response, which demonstrate an accurate, highly effective application of the relevant content knowledge and skills.
Support: Notice how the response provides limited evidence and examples or explanations, when provided, may be only partially appropriate. The response supports assertions with limited evidence from the exhibits provided. The strategy, rationale, progress monitoring, and extension activity are partially described. The explanations contain little or no evidence from the text or of relevant pedagogical knowledge. The explanation for the strategy's effectiveness is limited and reflects weak reasoning. The focus is not directly linked to Benjamin's specific academic need. It discusses motivation strategies without supporting that need with any evidence from the exhibits. The cross-curricular activity primarily focuses on real-world connections and not on how the activity specifically relates to extending Benjamin's knowledge of using multiplication to solve word problems. This differs from the score point "4" or "3" responses that provide relevant evidence, a higher degree of specific examples, and well-reasoned explanations supporting the major points in the assignment.
Performance Characteristics
The rubric created to evaluate your response to the constructed-response question is based on the following criteria:
| Completion | The degree to which the candidate completes the assignment by responding to each specific task in the assignment. |
|---|---|
| Application of Content | The degree to which the candidate applies the relevant knowledge and skills to the response accurately and effectively. |
| Support | The degree to which the candidate supports the response with appropriate evidence, examples, and explanations based on the relevant content knowledge and skills. |
Score Scale
The four points of the scoring scale correspond to varying degrees of performance.
| Score Point | Score Point Description |
|---|---|
| 4 | The "4" response reflects a thorough understanding of the relevant content knowledge and skills.
|
| 3 | The "3" response reflects a general understanding of the relevant content knowledge and skills.
|
| 2 |
The "2" response reflects a limited understanding of the relevant content knowledge and skills.
|
| 1 |
The "1" response reflects little or no understanding of the relevant content knowledge and skills.
|
| U | The response is unscorable because it is unreadable, not written to the assigned topic, written in a language other than English, or does not contain a sufficient amount of original work to score. |
| B | There is no response to the assignment. |
Return to Navigation